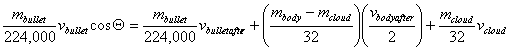
8. Plausibility analysis of the rearward lurch
The purpose of this section is to examine in simple terms whether it is plausible to consider further that a rearward lurch was created by recoil from the explosion of JFK's head. It is not necessary to prove that it WAS created, but only that it may reasonably have been. The answer is clearly yes. To reach this conclusion, we begin with the simplest possible arguments from physics and proceed through a series of progressively more complicated ones. The reader should keep in mind that the simpler the argument, the less reliable it may ultimately be. That is why we use a series of arguments.
The simplest plausibility argument
The simplest plausibility argument is that the incoming bullet contained
far more kinetic energy than the body ultimately showed in its rearward lurch.
Thus the bullet needed only to transfer a tiny portion of its energy in order to
create the lurch. This conclusion is easy to demonstrate. The kinetic energy of
the bullet, 0.5 mbulletvbullet2, is calculated
as follows:
KEbullet = 0.5(0.023 lb)(1800 ft s-1)2/32 ft s-2 = 1164 ft-lb
The incoming bullet contained 1164 ft-lb of KE. (Foot-pounds is the standard
unit of energy used in American ballistics.)
By contrast, the initial kinetic energy in the rearward lurch, 0.5 mtorsovtorso2,
is much less:
KEtorso = 0.5(85 lb)(0.8 ft s-1)2/32 ft s-2 = 0.85 ft-lb
The rearward lurch contained only 0.85 ft-lb of KE. That was less than 1/1000
the energy in the bullet (0.85/1164 = 1/1370)! The reason lies in the high speed of the bullet, which
enters as the square of the value. In kinetic energy, speed counts for much more
than mass. Light objects moving fast can easily have more kinetic energy than
heavy objects moving slowly. Thus the recoil energy of the body could have been
created by transferring only a tiny fraction of the energy from the bullet.
But this argument can be misleading, for at least three
reasons: (1) Other parts of the system receive part of the bullet's KE, such as
the two large fragments and the cloud. (2) In explosion like this, small masses
(the cloud and the fragments) tend to take a disproportionate share of the KE.
(3) Some of the kinetic energy is used as potential energy (energy of
deformation) to break the skull.
Nonetheless, there is so much excess kinetic energy that it is difficult to
argue that the bullet could not have created the lurch. Since these first estimates
cannot exclude this possibility, we move to the next stage of consideration.
Next-simplest plausibility argument
The next plausibility argument examines whether the large fragments
and the cloud, both of which exited to the front, have enough forward momentum
to stop the snap of the head and create the lurch. The approach is to estimate
the forward momentums of the cloud and the fragments from the Zapruder film and compare
them with the rearward momentums of the head and body, also derived from the film. If the
former two momentums are comparable to the latter two, it remains
plausible that fragments from the explosion could create the rearward lurch.
We do this in a quick step followed by a more-careful step.
The quick step forgets the forward fragments and focuses only on the forward
cloud. If its mass is 0.3 lb (out of 5–10 for the
head) and its forward speed is 500 ft s-1, the same as for the large
fragments (Section 3, Variables and Values), the
forward momentum of the cloud will be (0.3 lb)(500 ft s-1) = 150
ft-lb s-1. For an 85-lb body to have the same momentum rearward, it
would be moving at 150 ft-lb s-1/85 lb = 1.76 ft s-1, or
twice as fast as the observed 0.8 ft s-1. Thus the cloud seems to
have enough momentum to create the lurch, even allowing for a considerable
off-axis component of motion.
Here is the more-careful version of this step. To assess the various momentums, we need to know the masses
of the objects, the distances they traveled between Z312 and Z313, and the times
they took to travel these distances. The distances are easy to estimate—the
head traveled forward 2.18 in, the cloud about 1 ft, and the two large fragments
about 6 ft. The masses are a little harder to estimate. The mass of JFK's head
has already been estimated at 7 lb or so; here we can be conservative and use 8
lb. The mass of his torso can be set at 85 lb, which is one-half his weight. The
mass of the two large fragments can be estimated at 0.04 lb from Lattimer's
dimensions (Section 5, Variables and Values).
The mass of the diffuse cloud is very hard to determine, and
in fact it cannot be known accurately. But we can take a reasonable guess,
starting from Lattimer's observation that 70% of the right hemisphere was
missing, and the reported weight of the remaining brain of 1500 g. That means
that the 1500 g represents 65% of the original brain. Thus the 35% blown out
corresponds to 35/65 of 1500 g, or 800 g, which is 1.78 lb. To be safe, we can
use a range of 0.5–2 lb blown out.
The times are trickier to determine. The 55 ms from the end
of Z312 to the end of Z313 represents the sum of four times, the delay from the
end of 312 until the bullet hit (tdelay), the time of transit of the
bullet through the head (ttransit), which can easily be estimated at 1
ms or less), the duration of the forward snap (tsnap), which we don't
know anything about a priori, and the duration of the lurch (tlurch),
which we also don't know anything about. If tdelay is small,
then
tsnap + tlurch = 55 ms – 1 ms = 54 ms
Since this way of estimating tsnap and
tlurch overestimates them, the speeds derived in this way will be
underestimates of the true speeds, i.e., conservative estimates.
Now for the sake of simplicity we can start by assuming that tfrag
= tcloud = tlurch. That means that:
vhead = (2.18 in/12 ft
in-1)/tsnap
vbodyafter (at the
center of mass) = (2.3 ft s-1)/2
vfragsafter = 6 ft/tlurch
vcloud ≥ 1 ft/tlurch (≥ because the tiny fragments will slow down as soon as they hit the air)
Preliminary estimates of tsnap and tlurch can provide a feeling for the momentum of the head, body, fragments, and cloud. If we assume a 50/50 distribution, then:
tsnap = tlurch = 27 ms
That gives:
vfrags = 6 ft/0.027 sec = 222 ft s-1
vcloud ≥ 1
ft/0.027 sec = 37 ft s-1
pfrag1 (momentum) =
(0.02)(222)(0.34) = 1.5 lb ft s-1
pfrag2 =
(0.02)(222)(0.77) = 3.4 lb ft s-1
pfrags total = 5 lb ft
s-1
pcloud = (0.05–2
lb)(≥37 ft s-1) ≥
(18–74) lb ft s-1
pfrags + pcloud
≥ 23–79 lb ft s-1
How do these momentums compare with the momentums of the head and body (the snap and the lurch)? We calculate them and see.
vsnap = (2.18 in/12 ft in-1)/0.027 s =
6.73 ft s-1
vbody (at center of mass) = 0.4 ft s-1
psnap = (8 lb)(6.73 ft s-1) = 54 lb ft s-1
plurch = (85 lb)(0.4 ft s-1) = 34 lb ft
s-1
The momentum of the fragments, pfrags = 5 lb ft
s-1, cannot stop the snap or create a lurch (because 54 and 34 units
would be required). The momentum of the cloud, however, pcloud ≥
18–74 lb ft s-1, can stop the head and may also be able to create
the initial lurch, depending on how great its value (the cloud's) really is. In other words, it
remains plausible that some level of lurch can be created by the bullet alone.
We now modify the distribution of time to 2/1, or tsnap
= 36 ms and tlurch = 18 ms. That gives:
vfrags = 6 ft/0.018 sec = 333 ft s-1
vcloud ≥ 1
ft/0.018 sec = 56 ft s-1
pfrag1 (momentum) =
(0.02)(333)(0.34) = 2.3 lb ft s-1
pfrag2 =
(0.02)(333)(0.77) = 5.1 lb ft s-1
pfrags total = 7 lb ft
s-1
pcloud = (0.05–2
lb)(≥56 ft s-1) ≥
(28–112) lb ft s-1
pfrags + pcloud
≥ 35–121 lb ft s-1
The momentums of the head and body become:
vsnap = (2.18 in/12 ft in-1)/0.030 s =
5.05 ft s-1
vbody (at center of mass) = 0.4 ft s-1
psnap = (8 lb)(5.05 ft s-1) = 40 lb ft s-1
plurch = (85 lb)(1.15 ft s-1) = 34 lb ft
s-1
The new momentum of the fragments, pfrags = 7 lb ft s-1, still is not enough to stop the snap or create a lurch (because 40 and 34 units would be required). The new momentum of the cloud, pcloud ≥ 28–112 lb ft s-1, can easily stop the snap and create a sizeable, even violent, lurch. Nearly any part of the possible range can create the observed lurch. Again the lurch can be created by the bullet alone. (Note how you don't have to know much physics to get this far.)
Third level of plausibility argument
We can now move to the third level of plausibility argument,
a series of increasingly complex actual calculations. These form the rest of
this document. All we do here is outline their physical underpinnings.
There is only one rigorous way to calculate the physics of
the bullet-head interaction, and that is by simultaneously solving the equations
for the conservation of momentum and energy. This can be done in linear or
angular coordinates. We begin with the simplest form of the linear equations:
Conservation of X-momentum
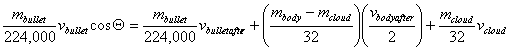
Conservation of total energy

A word about
units. These equations are set up to use the most natural form of data from the
JFK assassination. To that end, mbullet is in grains, but all the
other masses are in pounds. All velocities are in feet per second.
Two equations can be solved for
only two unknowns—all the other values must be supplied. Since more than two
values are always unknown here, some must be guessed at. The trick is to
identify the two most critical variables and solve for them by supplying values
for the others. Since we don't know the others' values, we must use a range of
possible values for each. The result gives a range of values for the two
critical variables, in tabular form that can also be used for a sensitivity
analysis similar to that done for the snap in earlier sections. I have developed
seven levels of complexity for the linear equations, ranging from very simple to
forms that require more than 30 variables. Each level has its own section, where
the equations are presented. I solved them in Mathcad, and have provided links
below to the Mathcad pages so that interested readers can experiment for themselves.
The idea is to keep the procedures accessible to as many readers as possible.
| Scenario | Description | Linear | Angular |
| Lurch 1 | Calculates from body, bullet, and cloud | L1L | L1A |
| Lurch 2 | Adds large fragments | L2L | L2A |
| Lurch 3 | Adds 3-D motion to cloud | L3L | L3A |
| Lurch 4 | Adds 3-D motion to large fragments | L4L | L4A |
| Lurch 5 | Adds 3-D motion to body | L5L | L5A |
| Snap/Lurch 6 | Adds four time intervals | SL6L | SL6A |
| Snap/Lurch 7 | Solves for PE instead of vcloud | SL7L | SL7A |
These Mathcad pages are the latest version (as of late February 2003), and contain four angular variables not otherwise discussed here. The earlier Θ is now Θv (for vertical). The corresponding horizontal angle for the bullet, Θh, has been added and given the default value of 9°. To allow for possible horizontal and vertical shifts of the axis of the cloud, Θclh and Θclv have been added. Default values of 0° have been entered for both.
Fourth level of plausibility
argument
The fourth level of plausibility is the angular analog to the
linear calculations in the third level. The simplest form of the angular
equations is:
Conservation of X-momentum

Conservation of total energy

The units of mass and velocity are the same as for the linear equations. The additional term fI is a constant of value 1.11. As with the linear equations, I have developed seven levels of complexity and solved them in separate Mathcad pages.
Results of the plausibility arguments
When all is said and done, the plausibility arguments show
that a WCC/MC bullet from Oswald's rifle can easily explain the forward snap.
That should come as no surprise. They also show that the bullet can easily explain
the initial part of the rearward lurch, via the phenomenon commonly known as
the "jet effect." The later frames of the lurch, during which the body
continues to accelerate rearward, must have come from some other
force(s), however, for the mechanical effects cannot drag out over several
frames. Careful analysis of the accelerations of JFK's torso seems to reveal
the effect of gravity on the torso as soon as it begins to lean backwards. But some other force interposes itself between the first
part of the lurch (where the jet effect is active) and the later pull of
gravity. This force is quite large and long-lasting. Independent calculations in one of the later
sections show that only a few percent of the bullets available at that time
could have created the
lurch directly (from a forward hit from the knoll). Only one reasonable
force is not ruled out by these calculations—a
neuromuscular reaction, or stiffening of the back, shortly after the impact to the head.
This must remain the working hypothesis until something better comes along,
which it hasn't in 39 years. Improbable? Yes. Impossible? No.
Ahead to
Lurch 1 Linear
Back to Snap Angular
Back to Physics of the Head Shot